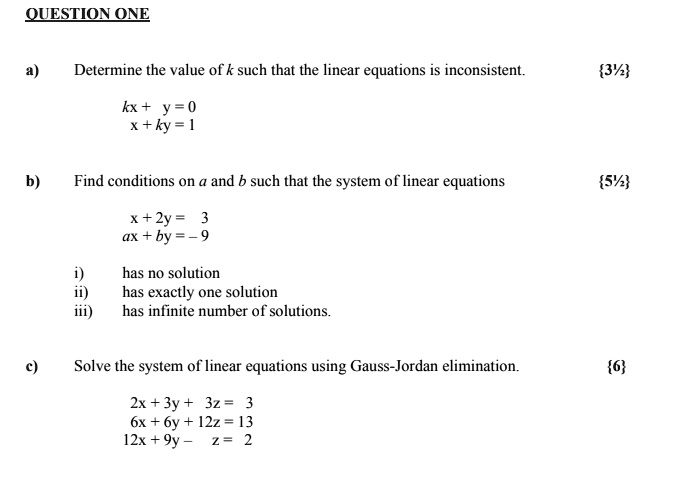
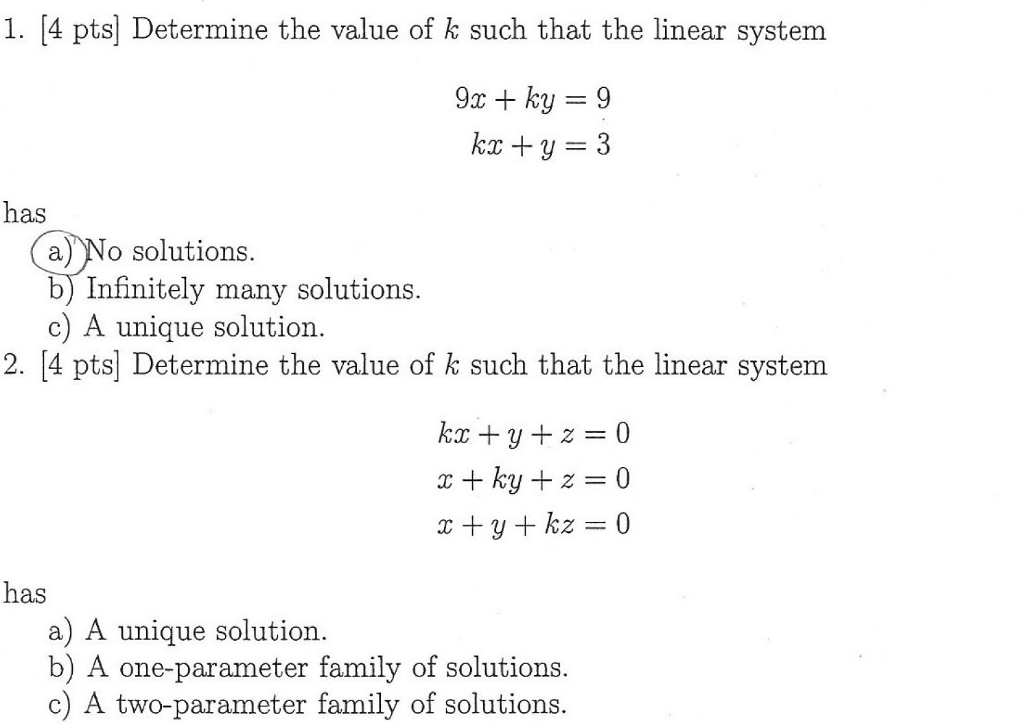
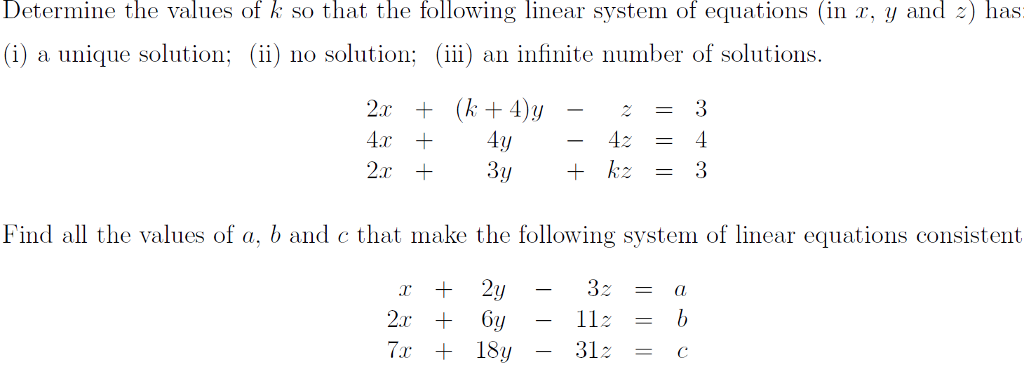
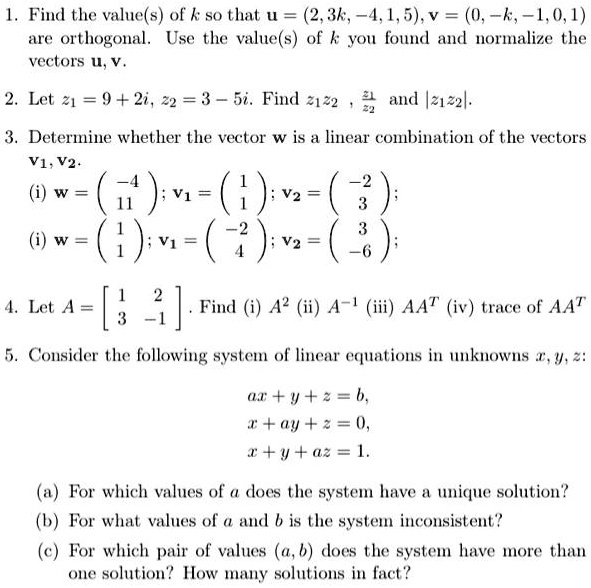
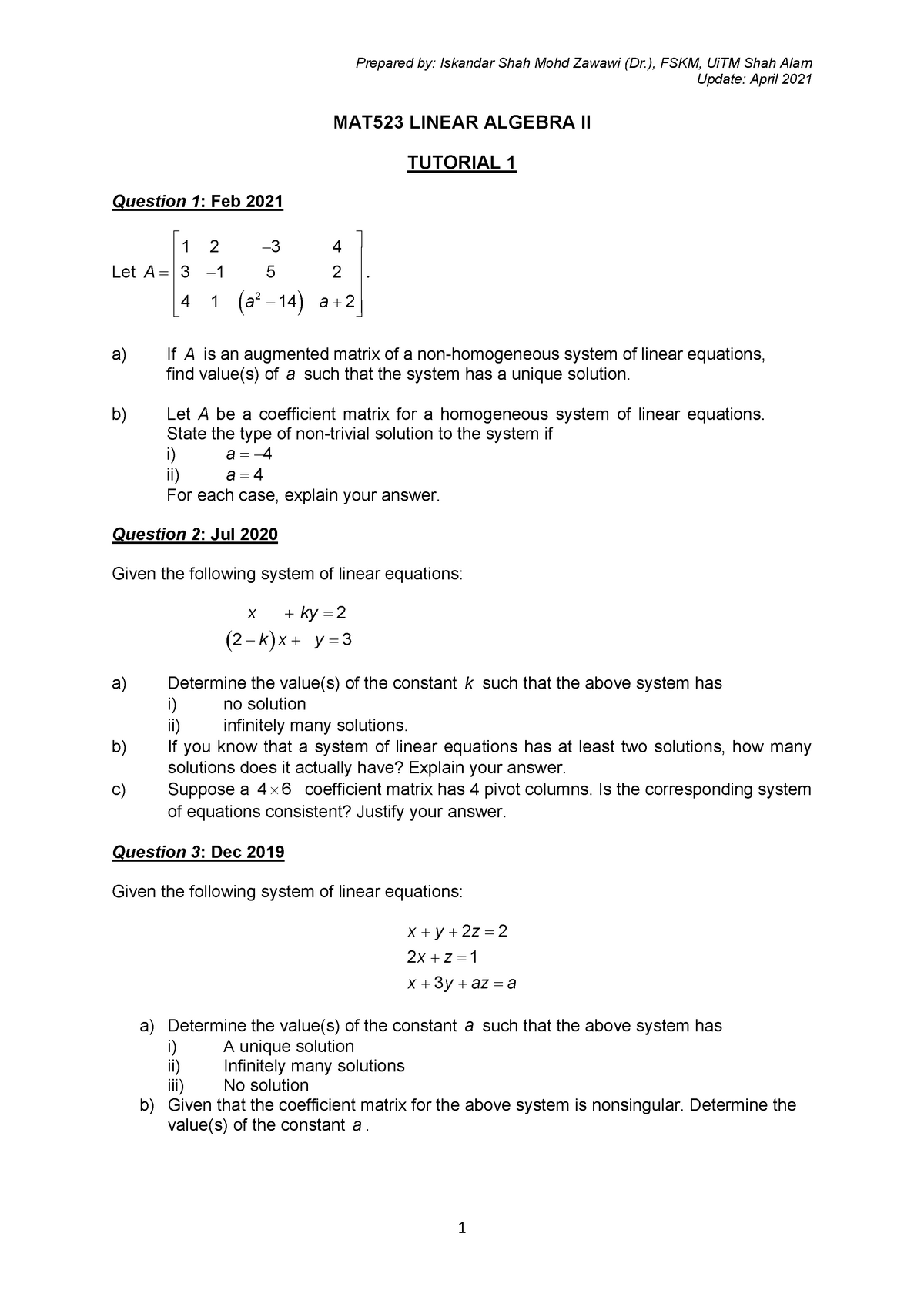
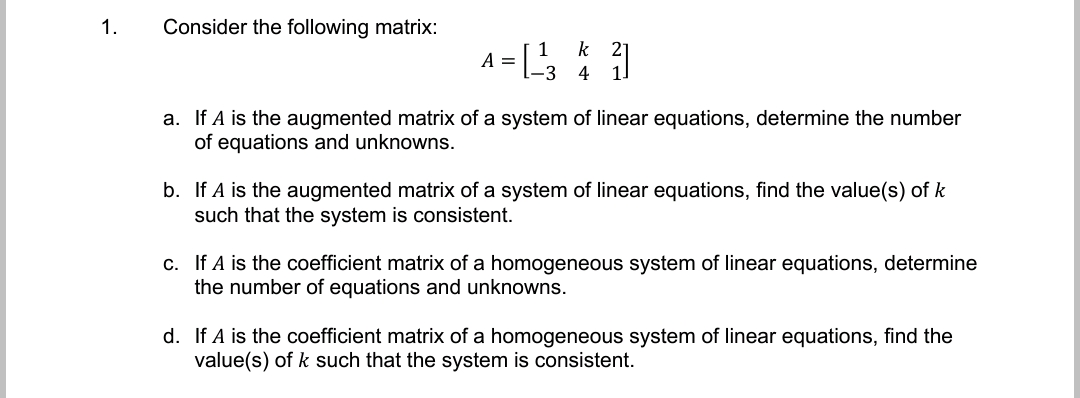
Find The Value Of K Such That The System Of Linear Equations Is Inconsistent
Solved Find The Value Of K Such That The System Of Linear Chegg Com
Find the value of k such that the system of linear equations is inconsistent. Find the values for k that make this system. So the system of equations is consistent one solution if h neq 2. Find the value of k such that the system of equations is inconsistent.
As pointed out by Christiaan Hattingh theres trouble if h 2 for any value of k. Get my full lesson library ad-free when you become a member. We have four X minus eight.
Okay so this problem eyes a little bit different. Enter your answers as a comma-separated list Infinitely many solutions he indicated number of solutions. Ive learned to find the solutions to linear systems using Gaussian Elimination.
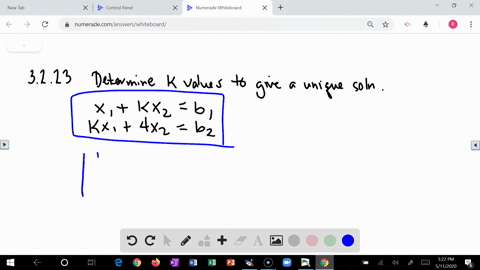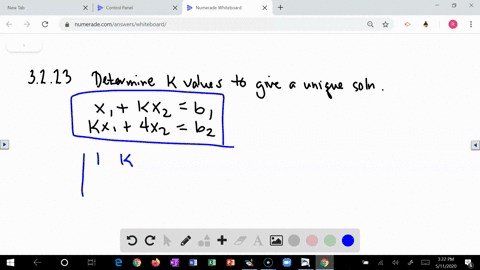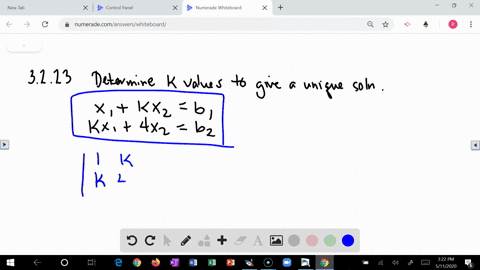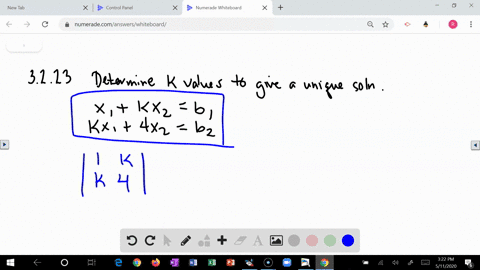
Determine the values of k such that the system of linear equations has the indicated number of solutions. -10y ky 9. Thus we get the equivalent system of equations as x 2 3 3 y 3 z 0.
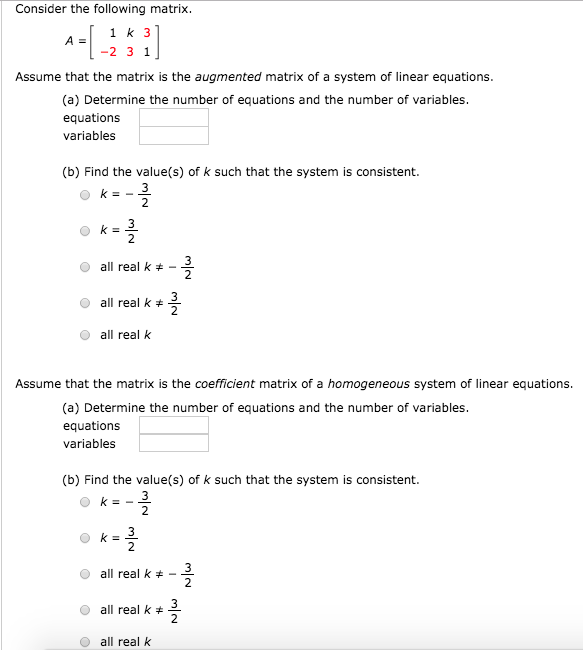
So inconsistent means uh for for the sake of what were looking for here is that these two lines have the same slow or that these two lines of parallel So for these two. You can form the augmented matrix for this system reduce it to echelon form and find out that k should be equal to -3. Solve if you can.
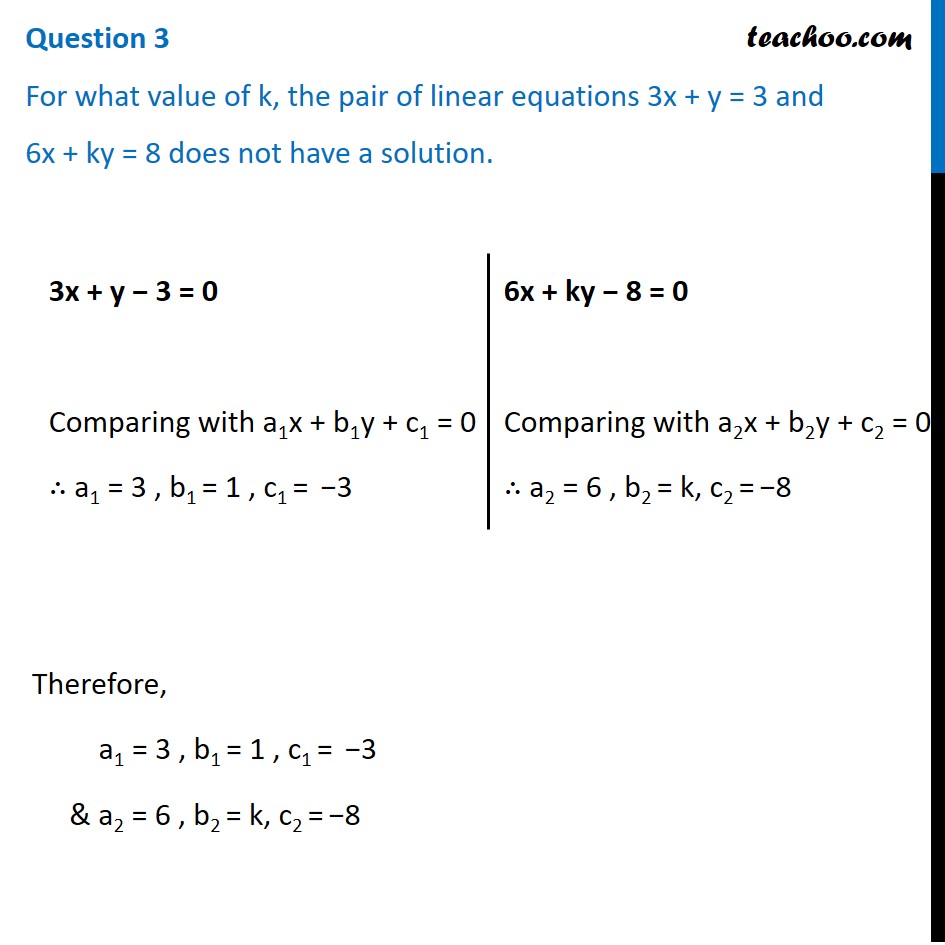
That means that in finance solution happens when von equation is just a multiple off other. Students can solve NCERT Class 10 Maths Pair of Linear Equations in Two Variables MCQs with Answers to know their preparation level. 15x- 3y 6.
In Exercises 71 and 72 find the value of k such that the system of linear equations is inconsistent. Leftbeginarrayl15x 3y 6-10x ky 9endarrayright.
Answered Determine The Values Of K Such That The Bartleby
Solved I The Determine The Values Of K Such That System Chegg Com
Solved Find The Value Of K Such That The System Of Linear Chegg Com
Solved Question One Determine The Value Of K Such That The Linear Equations Is Inconsistent 3 2 Kx Y 0 X Ky Find Conditions On And B Such That The System
Determine Unknown K For Linear System To Have Many Solutions Inconsistent System Youtube
Solved Determine The Value Of K Such That The Linear System Chegg Com
Choose H And K Such That The System Has A No Solution B A Unique Solution Youtube
Solved 3 Determine The Values Of K Such That The System Of Chegg Com
Solved Determine The Values Of K So That The Following Chegg Com
Solved Fiud The Value S Of K S0 That U 2 3k 4 1 5 V K 1 0 1 Ae Orthogonal Use The Value S Of K You Found And Normalize The Vectors U V Let 9
Solved Determine The Values Of K So That The Following Chegg Com
Answered Determine The Values Of K Such That The Bartleby
Choose H And K Such That The System Has A No Solution B A Unique Solution Youtube
Find Values Of K For Which Kx Y K 2 And X Ky 1 Have Infinitely
Solved Equations Variables B Find The Value S Of K Such That The System Is Consistent 5 5 All Real K All Real K All Real K Assume That The Matrix Is The
Find K From Augumented Matrix For No Solution Of Three Equations Youtube
Solved Consider The Following Matrix 2 3 1 Assume That The Chegg Com
Solutions To Systems Of Equations Consistent Vs Inconsistent Video Khan Academy
Solution Find The Value Of K Such That The System Of Equations Is Inconsistent 15x 3y 6 10y Ky 9
For What Value Of The Following System Of Equations Will Be Inconsistent 4x 6y 11 0 2x Ky 7 0 Sarthaks Econnect Largest Online Education Community
For What Value Of K The Pair Of Linear Equations 3x Y 3 And 6x Ky 8
Answered Find The Value Of K Such That The Bartleby
Determine The Values Of K Such That The System Of Linear Equ Quizlet
1
Find The Value Of K Such That The System Of Linear Equations Is Inconsistent 6x 2y 10 9x Ky 14 Brainly Com
Solved Find The Relation Between H And K Or All Possible Chegg Com
Solved Express The Column Matrix B As A Linear Combination Of The Columns Of A Use A1 A2 And A3 Respectively For The Columns Of A 1 6 10 A Course Hero
Solved Consider The Following Matrix K 3 A 2 3 1 Assume That The Matrix Is The Augmented Matrix Of System Of Linear Equations A Determine The Number Of Equations And The
Oneclass 43531 1 K Asl 231 Assume That The Matrix Is The Augmented Matrix Of A System Of Linear Equa
Solution Find The Value Of K Such That The System Of Linear Equations Is Inconsistent 6x 2y 8 Amp 8722 9x Ky Amp 8722 11
22 Find The Value Of K So That The Following Pair Of Linear Equations Is Inconsistent N 3 X Y 1 Text And 2 K 1 X K 1 Y 2 K 1
Rd Sharma Solutions For Class 10 Chapter 3 Pair Of Linear Equations In Two Variables Exercise 3 5 Avail Free Pdf
Find The Value Of K Such That The System Of Linear Equations Is Inconsistent Homeworklib
For How Many Values Of K Does The Given System Of Equations Have Infinitely Many Solutions Youtube
Tutorial 1 Solutions Pdf
Systems Of Equations Solver Wolfram Alpha
The Value Of K For Which The System Of Equations 3x 5y 0 And Kx 10y 0 Has A Non Zero Solution Is
Example 16 For What Values Of K Will The Pair Of Linear
Solved Determine The Value Of K Such That The System Of Linear Equations Is Inconsistent Note Course Hero
Solved In Exercises 19 And 20 Choose H And K Such That The System Has A No Solution B A Unique Solution And C Many Solutions Give Separate Answers For Each Part Begin Aligned
Find K For Which The System X 2y 3 And 5x Ky 7 0 Is Inconsistent
Document Mat523 Group Work Prepared By Iskandar Shah Mohd Zawawi Dr Fskm Uitm Shah Alam Studocu
Answered If A Is The Augmented Matrix Of A Bartleby
Find All Values Of K Such That The System Has No Solution Mathematics Stack Exchange
Expert Answer Find The Value Of K For Which The System Of Equations X 2y 3 And 3x Ky 1 Has A Unique Brainly In
Find The Value Of K For Which The Following System Of Linear Equations Has Infinite Solutions X K 1 Y 5 K 1 X 9y 8k 1
Math1131 Linear Algebra Chapter 4 Problem 17 Youtube
Solving Systems Of Linear Equations Harder Example Video Khan Academy
1
Get my full lesson library ad-free when you become a member.
Answer by Fombitz 32379 Show Source. 4k6k13 This system of linear equations can have infinite solutions only if the determinant of the 2xx2 matrix 4kk1 is 0. Answer by Fombitz 32379 Show Source. Thus we get the equivalent system of equations as x 2 3 3 y 3 z 0. Or 2 0 k 1 1 3 2 k 0 or 3 3 2 k 0 k 3 3 2 Putting the value of k the equations are x 2 3 3 y 3 z 0 1 3 x 2 3 3 y 2 z 0 2 2 x 3 y 4 z 0 3 Mutliply 1 by 3 and subtract from 2 and similarly multiply 1 by 2 and subtract from 3. Determine the value of k such that the following system of linear equations has infinitely many solutions and then find the solutions. What is the value of ab whole square minus a-b whole square. Also find all the solutions of the system for that value of k. With infinite solutions begin cases kx y z 1 x ky z 1 x y kz 1 end cases.
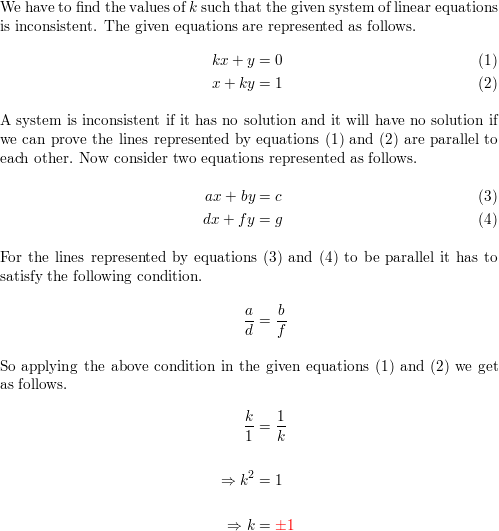
Okay so deal system awfully near a question has infinitely many solution when the same line is represented by the two equations. Um two equations are inconsistent. Inconsistent no solutions With unique solution. Solve if you can. Linear algebra - Value of k for whichthe system is inconsistent - Mathematics Stack Exchange. Or 2 0 k 1 1 3 2 k 0 or 3 3 2 k 0 k 3 3 2 Putting the value of k the equations are x 2 3 3 y 3 z 0 1 3 x 2 3 3 y 2 z 0 2 2 x 3 y 4 z 0 3 Mutliply 1 by 3 and subtract from 2 and similarly multiply 1 by 2 and subtract from 3. Leftbeginarrayl15x 3y 6-10x ky 9endarrayright.
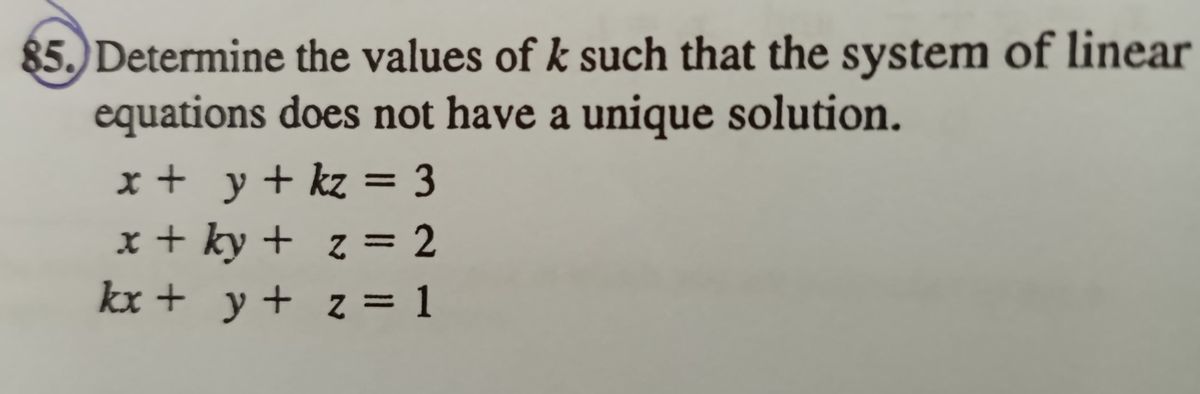
Post a Comment for "Find The Value Of K Such That The System Of Linear Equations Is Inconsistent"